Infinity

This paper asks why so few philosophers and scientists have turned their attention to the idea of infinity, and it expresses surprise at the readiness with which infinity has been accepted as a meaningful concept even in fields where it causes serious logical difficulties. I try to show that belief in a universe containing an infinite number of material objects is logically flawed, and I examine the cosmological consequences.

THE CONCEPT OF INFINITY

The idea of infinity arises in several different contexts. Most of the applications to which we shall refer in this paper belong to one or other of the following six categories:

1. The sequence of natural numbers, 1, 2, 3, ..., is said to be infinite.
2. In geometry, the number of points on a line is said to be infinite.
3. In mathematics, many examples occur of sequences of numbers which tend to infinity.
4. It is often assumed that time is infinite, in the sense that it had no beginning, or will have no end, or both.
5. Likewise, space is often assumed to be infinite in extent.
6. Some theories of cosmology suppose that the amount of matter in the universe, i.e. the number of stars or of atomic particles, is infinite.

Modern astronomers do not agree on whether or not the universe is infinite in extent. While books on cosmology display much detailed knowledge of the history and structure of the universe, they appear to find the issue of infinity difficult to decide. Mathematicians tell us that the question is closely related to the average curvature of space, and everything depends upon whether this curvature is positive, zero or negative. If it is positive, we are told, the volume of space is finite, but if it is zero or negative the volume must be infinite, and this is usually taken to imply that the number of stars and galaxies must also be infinite. In fact this curvature is very close to zero, making it difficult to determine by observation or measurement which sort of universe we live in. Many of the formulae in cosmology must therefore be given in three different forms, so that the correct version can be chosen when we do eventually discover whether the value of k is +1, 0 or -1.

The question of the finiteness of time seems equally uncertain; most cosmologists now believe that the universe began with a big bang, all its material content coming into being at a single point in a colossal explosion, and with time itself beginning at this first moment. But opinion is divided on whether it will end with some sort of "big crunch", with everything finally ceasing to exist in a mammoth implosion.

It is surprising that cosmologists do not concern themselves greatly with these questions of finiteness. They give us the three formulae, corresponding to the three possible values of k, and leave it at that. Indeed some books on the subject fail to state clearly whether particular arguments apply to an "open" or a "closed" universe, as if it did not really matter. Some have no reference to "infinity" in their index. Surely few questions are more significant than whether the universe is finite or infinite.

At one time there seemed to be a strong argument against the number of stars being infinite. A simple calculation shows that if it is, then the whole night-sky should be ablaze with light. The surfaces of the stars are, on average, as bright as the surface of the sun, and if they are infinitely numerous it can easily be shown that any line of sight will eventually terminate on a star, so that the whole sky will shine as brightly as the sun. It can be argued that the most distant stars might have their light dimmed by passing through gas or dust on its way to us, but if this were the case, the gas itself would be raised to such a temperature that it too would shine with this same brilliance. This problem was known as "Olbers' Paradox", after Heinrich Olbers (1758-1840). But it has now been resolved; even if the universe were infinite, we know that its expansion would provide an explanation for the darkness of the night-sky. Distant stars are dimmed not because of intervening matter, but because they are moving away from us, and the wavelength of their light is increased, and its energy reduced, by this motion. So Olbers' effect does not now present an obstacle to those who believe in an infinite universe. But here again it is surprising that taking the number of stars to be infinite is an assumption that can be adopted or discarded at pleasure, without considering whether it should be ruled out on logical grounds.

MATHEMATICIANS' INFINITY

The idea of infinity has been used increasingly over the centuries by mathematicians, and in general they have been more circumspect than have astronomers in their use of the word. The natural numbers clearly have no upper limit; the process of counting the numbers can never be completed. And if I name a number, however large, you can always name a larger one. So we can agree that the class of numbers is unlimited. We might even allow the class to be called "infinite". But it is a mistake to say that the number of its members is "equal to infinity" for infinity cannot itself be a number. It can be defined only as something "greater than any number we can name".

Modern mathematicians make much use of "infinite sequences" of numbers, but during the nineteenth century they carefully defined their concepts in this field to avoid using the word "infinity" as if it were indeed a number. To take a simple example, if a sequence is defined so that the nth term is 1/n, we get the values 1, 1/2, 1/3, 1/4, ... as n takes the values 1, 2, 3, ... The fractions get closer and closer to 0, but never quite reach it. We disallow the statement "the nth term of the sequence equals 0 when n equals infinity", but instead we say "the limit of the sequence is 0 as n tends to infinity". This is then carefully defined as follows: "For any quantity q, however small, there exists a value of n such that every term of the sequence after the nth differs from 0 by less than q". This is perfectly explicit, and makes no reference to the number infinity. Likewise, when considering continuous variables, we disallow the shorthand form "1/0 equals infinity", and we say rather, if y = 1/x, then "y tends to infinity as x tends to 0", and this is rigorously defined to mean "For any quantity Q, however large, there exists a value of x such that y is greater than Q whenever x is numerically less than this fixed value". In this way modern mathematics allows us to discuss infinite sequences in a way which is logically sound.

But other branches of mathematics have found it necessary approach the concept of infinity more directly, and to adopt the notion of a "completed infinity", and as a result have become dogged by paradox. They begin by defining carefully what is meant by two numbers being equal; if the elements of two classes of objects can be put into one-to-one correspondence with each other, then they must contain equal numbers of elements. If every seat on an aircraft is occupied by a passenger, and every passenger has a seat, then the number of passengers must equal the number of seats. But difficulties arise when this definition is applied to unlimited classes such as the natural numbers; here is a simple example of such a paradox. Mathematicians use the symbol "Aleph-0" to represent the infinity we would obtain if we could count all the natural numbers. But because every number can be multiplied by two, the natural numbers can be put into a one-to-one correspondence with the even numbers, as the following lists show:

   Natural Numbers   1  2  3  4  5  6  ....
   Even Numbers      2  4  6  8  10 12 ....

It follows from this that the number of even numbers must also be equal to Aleph-0, according to this one-to-one definition of "equality". What sort of a collection is it that still contains the same number of elements after we have removed half of them? And what sort of a number (apart from 0) is equal to twice itself?

The paradoxes become even more perplexing when we consider fractional numbers, such as 1/2, 5/8 or 999/1000. It is clear that the number of fractions between 0 and 1 must be unlimited, for it is always possible to find a new fraction between two given fractions, however close together they are.

To satisfy the curious, this can be done simply by adding the tops and adding the bottoms; thus 5/17 lies between 2/7 and 3/10.

The ancient Greeks realised that fractional numbers are needed in order to describe the lengths of lines, or to indicate the position of a point on a line. Because we can always find a fraction between any given pair of fractions, it follows that the complete set of fractions has no gaps in it. So the class of all fractions would seem to be sufficient to express accurately the length of any line. This was accepted by the Greeks more than 500 years B.C., and they were surprised to discover that it is just not true. If a square has sides of length one inch, then Pythagoras' well known theorem shows that the length of the diagonal must be the square root of 2, and Pythagoras himself proved that this number is not, in fact, a fraction.

His proof was a remarkable achievement in its day, but is not difficult to comprehend today. Suppose the fraction m/n does equal the square root of two, and suppose it is in its lowest terms, i.e. it does not need cancelling. Then its square must equal 2, i.e. m squared over n squared equals 2, and so m squared is twice n squared. This shows that m squared is an even number, and so m is even, and m squared must divide by 4. It follows that n squared must divide by 2, and so n also must be even. But this contradicts our stipulation that m/n does not need cancelling, and the contradiction shows our original supposition to be false; the square root of 2 cannot be a fraction.

So the class of fractions, although it contains no gaps, is not adequate for describing the lengths of lines. For some lines we need also numbers such as the square root of two, which cannot be expressed as fractions. Until quite recently this presented an intriguing puzzle. But what seems to be overlooked, even today, is that when we deal with the real world of geometrical figures, fractions are sufficient for specifying the length of any line we have measured. Measurements necessarily must be made to some limited degree of accuracy, depending on our method and the care we take. However small the inaccuracy we will allow, the length of a line can be expressed as a fraction (or what amounts to the same thing, a decimal) to whatever precision our measuring technique will allow. The length of the diagonal of an ideal square may indeed be "irrational", but this is of no concern to us when we measure the diagonals of real squares. No measurement of a square's diagonal could prove that its actual length is irrational.

Undeterred by the paradoxes they were unearthing, mathematicians in the nineteenth century studied the infinite numbers in detail. They accepted it as true, despite the apparent contradiction, that the number of even numbers was indeed the same as the number of all the natural numbers. As we have seen, the number of fractional numbers then turns out to be the same again, and they began to suspect that all infinite numbers must equal each other. So it was a surprise when Cantor (1845-1918) showed that the class of real numbers, which includes also the irrationals, contains a larger infinity than Aleph-0. The criterion he used was again to ask whether this class could be put into one-to-one relationship with the natural numbers, in other words whether it could be counted. The set of even numbers, or the the set of fractions, are said to contain Aleph-0 members because they can be counted (in theory, although we could never end the process), while the class of real numbers cannot. So Cantor introduced us to a whole heirarchy of infinite numbers, Aleph-0, Aleph-1, ..., which are not equal to each other.

INFINITY IN THE REAL WORLD

The world of the Pure Mathematician is far removed from the real world. In the real world there is no difficulty finding the length of the diagonal of a square and expressing this as a decimal, but in the perfect world of Pure Mathematics this cannot be done. In the real world we know the process of counting the natural numbers can never be completed, so that the number of numbers is without meaning, while the mathematician finds it necessary to say that if the process were completed, the number would be found to be Aleph-0. These are harmless follies; what the mathematician gets up to inside his ivory tower need not concern those outside.

But the mathematician's ideal world did impinge on reality at the beginning of the twentieth century when Russell (1872-1970) attempted to reduce all mathematical reasoning to simple logic. Even the natural numbers themselves could be defined in terms of a simpler concept, but to make this possible Russell found it necessary to assume that the number of real objects in the universe is itself infinite. Here again I find it astonishing that he made this assumption so glibly. He called the principle his "Axiom of Infinity". Now an "axiom" is something which is self-evident, unlike a "postulate", which is assumed for convenience even though it is not self-evident. Why did Russell not refer to the principle as his "Postulate of Infinity"? To me it is far from self-evident that there are an infinite number of things in the universe; in fact I cannot see that the statement has any meaning. Infinity is an indispensible concept in Pure Mathematics, but is it not meaningless when applied to the number of real things? Nature, however, retaliated for Russell's cavalier assumption that this number is in fact infinite. The theory of classes which he developed from this assumption eventually showed some unacceptable inconsistencies which he could avoid only by making arbitrary restrictions on the types of class which the theory would allow. To my mind this represented an elaborate reductio ad absurdum proving that the number of objects in the universe can not be infinite.

There is another strong argument that to talk of a universe containing an infinite number of particles is without meaning. It has often been pointed out that, in such a universe, anything which can exist without trangressing the rules of nature, must of necessity exist somewhere, and anything which can possibly happen, will happen, So there must somewhere be another planet which its inhabitants call "Earth", with a country called "England" whose capital is "London", containing a cathedral called "St. Paul's". Indeed there must be an infinite number of such planets, identical in every respect except that the heights of these copies of St. Paul's differ among themselves. Is this not sufficiently ridiculous to convince believers in an infinite universe that they are wrong? Is not this a further indication that to talk of an infinity of material objects must be meaningless?

As we have shown above, mathematicians have tried to avoid the use of infinite magnitudes wherever possible. They have succeeded in deflecting such ideas as series which "tend to infinity", or the number of points on a line being "equal to infinity", by re-stating propositions of this sort in terms which do not require use of the word "infinite". Cantor and others were not able to treat in a similar fashion their discussion of the equality or inequality of the different infinities they had discovered, but it must be conceded that their arguments had no relevence in the real world of material particles and real magnitudes. Indeed, their discussion of the various "orders of infinity" which they introduced into Pure Mathematics, Aleph-0, Aleph-1 and so on, is no more than a fascinating game whose rules, such as that defining "equality" in terms of one-to-one relationships, are man-made and arbitrary; a different definition of equality would result in a different pattern of relationships between the various infinities. Only when attempts are made to relate the concept of infinity to objects in the real world, as Russell did with his Axiom of Infinity, do we meet insurmountable logical contradictions.

COSMOLOGY

Our reasoning seems to lead to the conclusion that the number of particles in the universe is finite, but it has nothing immediately to say about the total volume of space or the total duration of time. Physical quantities such as space and time can be quantified only by constructing some system of measurement, whereas no such system is required to enumerate entities such as particles. It is the process of counting which we maintain must terminate when applied to real objects in the universe; we are not asserting that the whole of space must be limited in extent, or that time must have a definite start and finish.

Indeed a process of measurement can sometimes lead to one quantity being described as infinite in extent, while another equally valid process can result in this same quantity having a finite magnitude. As an example, suppose a very long straight railway line is spanned by a bridge, and a surveyor standing on the bridge wishes to assess the distance along the track to a stationary wagon. He could do this by coming down from the bridge and laying a measuring rod along the track until he reaches the wagon. He would describe the distance as so many metres. But alternatively he could determine the distance by measuring the angle between a vertical plumb line and his line of sight as he observes the wagon from the bridge. The further away the wagon, the greater would be this angle, and he could use its value to calculate the wagon's distance in metres. But if he wished he could describe the distance by quoting this angle itself, to give the wagon's distance as so many degrees. Although in most situations this would prove less convenient, both measures are acceptable, for each identifies an exact point on the track, and they both increase steadily the further away is the wagon. But if, by some miracle, the track were infinite in extent, as the wagon receded the number of metres would tend to infinity, while the number of degrees would merely approach 90^o. The conclusion to be drawn from this paragraph is not that there are better ways of measuring distances on a railway than those we currently adopt, but that there is no intrinsic difference between a finite and an infinite distance, for some method of measurement must be stated or implied, and the mere convenience of that method cannot be taken as determining the finiteness or otherwise of a length.

It would seem a meaningless question, therefore, to ask whether the amount of space in the universe is finite or infinite, for equally valid methods of measurement could give conflicting answers. But if we allow ourselves to be influenced by the convenience argument, some methods are certainly preferable to others. Here on earth we make much use of rigid bodies, such as tables, bricks, and measuring rods, and it is fortunate that in one system of measurement the dimensions of all such bodies appear not to change unless they are subject to extreme forces or processes, so it is not surprising that this is the system we adopt. The stars are not rigid in this sense, but with this same measurement system a star is very nearly spherical. And the diameters of stars of similar types are all approximately equal, so we adopt this system, as far as possible, for defining astronomical sizes and distances. The complexities of General Relativity, and the expansion of the universe, present new difficulties when describing the dimensions of the universe as a whole, but we shall continue to discuss whether or not space is finite without too much risk of ambiguity, while constantly bearing in mind that we are not talking about an intrinsic property of the universe, in contrast with our discussion of the number of objects it contains, which is an intrinsic property. In such discussion we shall assume a convenient system of measurement, a system in which rigid bodies and stars preserve their dimensions even if transported to remote regions of space or time.

It is easy to understand the pressures that existed in earlier times to believe that the universe must be infinite. In past centuries it was thought the only alternative to an infinite volume was one which had a boundary, some sort of screen which would loom up in front of anyone who had travelled far enough. Both these beliefs seemed implausible, but the infinite universe was the lesser of the two evils. This dilemma forms the subject for the first of Kant's well-known "antimonies". He argues that pure reason leads inescapably to the conclusion that space and time are finite, and equally inescapably to the conclusion that they are infinite. He offers no solution to this contradiction. But in recent years we have not needed to make a choice between these two unpalatable beliefs. Gauss and Riemann demonstrated in the middle of the nineteenth century that Euclid's is not the only possible geometry, and that there is no reason why the geometry of our universe should necessarily obey Euclid's rules. And some years later, Einstein showed that our space indeed does not abide by them. It is the deviations from Euclidean geometry which explain gravitation, and these deviations, although very small in the part of space we can explore directly, have been detected experimentally. So now that we are freed from the shackles of Euclidean geometry, there is no difficulty in reconciling a finite space with the absence of any boundary. We cannot actually visualise a three-dimensional space or a four-dimensional space-time which is finite but nevertheless unbounded, but we have a perfect two-dimensional analogy when we picture the surface of a sphere. The surface of a globe, for example, has no boundary in the sense that a country has a boundary, and yet its area is strictly finite and calculable. Einstein's General Relativity does not decide for us whether in fact our universe has this form, but if it has, then the Kantian contradiction vanishes. Neither theory nor observation has yet proved adequate to decide conclusively whether our universe is of this type, but Einstein believed strongly that it is, and that the world we live in is indeed finite, but has no boundary.

Throughout most of the twentieth century it was known that the universe is expanding. Edwin Hubble discovered in the 1920s that the galaxies are moving away from each other, and the greater the distance of a galaxy the faster is it receding from us. And almost until the end of the century it was assumed that this expansion must be slowing down, because of the gravitational attraction between the galaxies. What was not so certain was whether this retardation would be strong enough eventually to reverse the expansion, or whether, because gravity grows weaker as distances increase, the universe would continue to expand, ever more slowly, but for ever. It will be realised from the picture painted in the previous paragraphs that I myself believe the former of these two possibilities must come about; the expansion must reverse at some distant point in time, leading to a big crunch, and ensuring that the length of life of the universe remains finite.

Now just as the surface of a sphere provides a good analogy for a finite but unbounded three dimensional universe, but with one dimension missing, it provides an equally good analogy in two dimensions for the four dimensions of space-time representing the complete history of such a universe, provided this history is of finite duration as I am suggesting. We can consider each circle of latitude to represent the whole of space at one particular time (but with two dimensions missing), and each of the meridians to represent time, and we can take the North Pole as its first moment and the South Pole as its last.

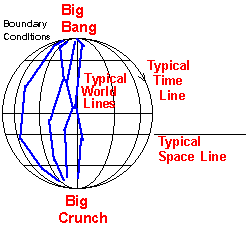
Translating this picture back to our usual viewpoint, we see our three-dimensional universe coming into existence in the big bang, (at the North pole of our spherical analogy), expanding to a maximum volume over some tens of billions of years, and then collapsing into the big crunch at the South pole. The picture has a satisfying symmetry which, in the absence of any real evidence to the contrary, makes it pleasing and plausible.

(The argument of the previous paragraphs has been severely condensed, because a much fuller treatment will be found in Chapter 2 of the author's book "Quantum Physics: The Nodal Theory" on another part of this website. The reader is earnestly requested to read Chapter 2 now, and then to read again the present paragraphs.)

RECENT DEVELOPMENTS

What was not expected was the announcement in 1998 by a group of astronomers, led by Saul Perlmutter of Berkeley University, that they had reason to believe the expansion was not slowing down at all, but was actually accelerating. Some stars end their lives as a "supernova", a gigantic explosion when for a few days they shine with a brilliance many billions of times that of the sun. So great is the light output from these events that with modern telescopes we can often see supernovae which occurred billions of light years away, and hence billions of years ago. And a particular class of supernovae, known as Type Ia, are known all to have almost identical intrinsic brighnesses, so that measuring their apparent brightness as seen from the earth can give an accurate determination of their distance. So Perlmutter and his colleagues carefully measured the distance of some distant supernovae using two different methods. By observing their spectra, and noting the "Doppler" shift in wavelength of their light due to the recession of the galaxies in which they were located, they could measure the distance of these galaxies using a well known and well tried formula. And by measuring their apparent brightness they could obtain these distances in another way, measuring, in effect, the length of time their light had been travelling to reach us. From these two measurements it is possible to estimate the acceleration of the region of space where they are located, and Perlmutter and his team found to their surprise that this acceleration had a positive value, rather than the negative value they expected.

Since 1998 several more Type Ia Supernovae have been observed, and evidence does seem to be accumulating that the expansion is increasing rather than decreasing in speed, and many scientists do appear to be convinced by this evidence. Such an acceleration must require a vast expenditure of energy, and no explanation has been agreed upon for the source of this energy. It has, however, been given a name, and space is supposed to be filled with this "dark energy", which manifests itself only in one way, by producing this acceleration of the distant galaxies.

My own view is that it is much too soon to reach conclusions. The whole investigation is fraught with great difficulty. Each supernova must be observed during the brief period that it shines with maximum brilliance so that accurate photometry can be performed, and it gives only a few days' notice that this is about to happen. The world's largest telescopes are in great demand; observing time is very valuable, and usually must be booked months in advance, making the observation of such ephemeral phenomena very difficult. But this is nothing compared with the theoretical difficulty of the calculations. We mentioned two ways of measuring distances in space, with the difference between the results forming the basis for the whole theory. There are at least four other ways of describing the distances of stars, and only a thorough understanding of cosmology and General Relativity can show which two of these will give the required results. And there is a multitude of corrections that must be applied to the figures before the tiny differences emerge which can distinguish between an acceleration and a deceleration; remember we are looking at events which occurred billions of years ago. Such tentative evidence is not yet sufficiently weighty to change the author's convictions that the universe must be finite in all respects.

Furthermore, cosmologists have been known to make mistakes. In the 1930s they used their knowledge of the distances of some neighbouring galaxies and their rates of recession to calculate the age of the universe, and the results seemed to prove that the sun and the earth were several times as old as the universe which contains them! Only in 1952 was it discovered that every galaxy was nearly three times as far away as had been believed, for a mistake had been made in calculating the distance of the nearest one. Then for several decades, when it became possible to estimate the total mass of a galaxy, they found that every one was rotating at the wrong speed. Only recently have they begun to understand the "dark matter" (not to be confused with the "dark energy" mentioned above) which forms an invisible part of each galaxy. When account is taken of the additional mass of this material the figures are no longer inconsistent. Then just a year after the apparent discovery of the "acceleration" of the galaxies, some members of Perlmutter's team issued a statement casting doubt on their conclusions, on the gounds that supernovae in the early universe appeared to have different characteristics from those observed nearer to home, and so may not have reached exactly the same maximum brilliance. Still more recently it has been decided that, even if we are now in a period of acceleration, this must have been preceded by several billion years of deceleration. Indeed, it is not now considered unreasonable that the acceleration might again be replaced by a deceleration some further billions of years down the line.

On these shifting sands it does not seem expedient to rebuild one's complete theory of cosmology, and I shall let the whole of this paper remain as it is until some more substantial foundations present themselves. In particular I remain convinced that the universe will end within a finite time, with some sort of big crunch marking its demise. I discuss the nature of this event in considerable detail in another essay on this website, and I do hope the reader will proceed at once to study The Collapse of the Universe and the Reversal of Time.